General Planar Motion with a Center of Mass Reference - Example Problem 6.7-3

 

When rockets rise to an sufficient altitude above the earth’s atmosphere, their aerodynamic control surfaces are no longer able to generate the lift forces necessary to maneuver the vehicle. One approach for steering rockets under these conditions is called thrust vectoring and involves gimbaling the rocket’s engine such that the direction of the thrust force can be controlled. This is the situation depicted in the figure of a 20,000-kg rocket directed 20 degrees away from vertical with its thrust vector gimbaled 1 degree away from the longitudinal axis of the rocket. If the rocket is experiencing 300 kN of thrust at an altitude where the acceleration due to gravity is approximately 8 m/s², determine the acceleration of the mass center G and the angular acceleration α of the rocket. The rocket has a centroidal radius of gyration of 5 m and the length L depicted is 10 m.

 

 

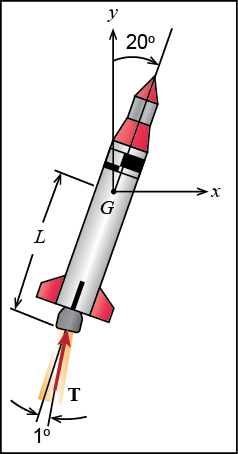
Given:

• m = 20,000 kg
• θ = 20^o
• φ = 1^o
• T = 300 kN
• g = 8 m/s²
• k_G = 5 m
• L = 10 m

 

Find: a_G , α

 

Video solution:

 

The following video walks you through the solution to this problem. It is suggested that you try solving the problem first and then, if you have difficulties with the solution, watch the video for help.

 

 

Interactive solution:

 

Which moment equation should we use?

 

∑M_G = 0
∑M_P = I_Pα + r_G/P x ma
∑M_G = I_Gα