Plane Curvilinear Motion - Polar Coordinates

 

Position

 

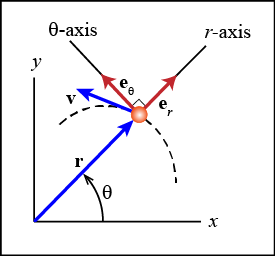
The polar coordinate system is defined by the coordinates r and θ. Just like the n-t coordinate axes, the r and θ axes are attached to and move with the particle.

 

• Position: (r) The vector that starts at the origin of the x-y coordinate system and points to the particle.

 

r = re_r

 

 

Velocity

 

The r-θ axes are body-fixed. That means that they are attached to and move with the particle. We know that velocity is the time rate of change of position as shown below. As you can see, the velocity equation contains the time derivative of a unit directions vector. The time derivative of a unit direction vector in a body-fixed coordinate system is generally not zero.

 

v = dr/dt = d(re_r )/dt = (dr/dt)e_r + r(de_r /dt)

 

de_r /dt = (dθ/dt)e_θ

de_θ /dt = -(dθ/dt)e_r

 

Using the values for the time derivative of the unit direction vectors, we get the following polar coordinate velocity equation.

 

v = (dr/dt)e_r + r(dθ/dt)e_θ

 

Acceleration

 

The acceleration is the time rate of change of velocity as shown below. Again, the derivatives of the unit direction vectors are not generally zero.

 

a = dv/dt = d((dr/dt)e_r + r(dθ/dt)e_θ )/dt

 

Using the above expressions for the time derivatives of the unit direction vectors, we get the following polar coordinate velocity equation.

 

a = ((d ²r/dt ²) - r(dθ/dt)²)e_r + (r(d ²θ/dt ²) + 2(dr/dt)(dθ/dt))e_θ